DeepEarthScience

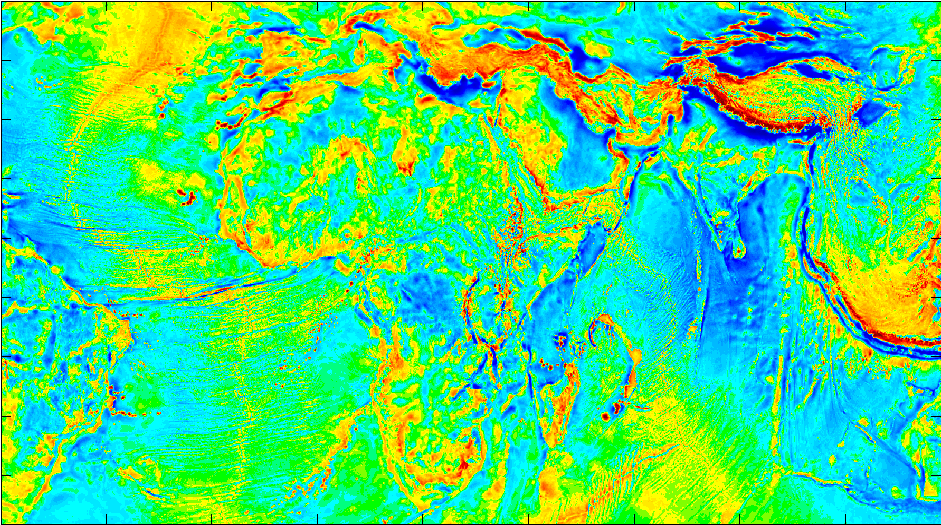
Last week I visited a conference which had the theme: "The Face of the Earth". At the conference were very inspiring talks and interesting scientists. It gave me new inspiration for my blog and my PhD research. It also gave me artistic creativity. So enjoy my art work titled: "The Face of the Earth viewed by a gravity scientist!" (click on figures to enlarge) The figure represents the free air anomaly of the Earth. This anomaly is the deviation of the gravity signal from the main ellipsoidal signal (the 9.81 m/s^2 you learned in high school). The colors represent the magnitude of the deviation. So more mass is red and blue means less mass then the main signal (green is zero). But you don't want to talk numbers, you want to enjoy the colors, because that is art all about. The above picture is of course the old continent Africa. You can clearly see some nice features in the gravity field. In the middle of Africa (Congo) is a large blue area, showing the l...

I need your help!  A few posts ago , I tried to measure the shape of the Earth using my acceleration chips in my macbook. After a quick sensitivity analyses I found out that the precision of the chips was not sufficient to do this exercise. However, I really want to see if I can measure the curvature of the Earth by looking at its gravity field. So instead of high-tech measurements with my macbook, I want to see if a low-tech solution (a string pendulum) is more accurate. The basic physics behind a pendulum measurement. The relationship between the period (T) of the pendulum and its length (l) and the local gravity (g) is (yes, high school again): Well, this is not entirely correct, but for this experiment (keep the amplitude low!!!) it is good enough (take a look  here  to get a better relationship). So, to measure the local gravity (g) we need to know the length of the pendulum (half-measure!!!) and the period of the pendulum. So, what is my set-up? A p...